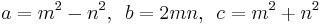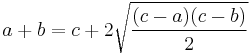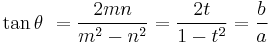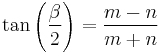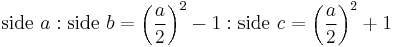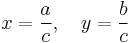Pythagorean triple

A Pythagorean triple consists of three positive integers a, b, and c, such that a2 + b2 = c2. Such a triple is commonly written (a, b, c), and a well-known example is (3, 4, 5). If (a, b, c) is a Pythagorean triple, then so is (ka, kb, kc) for any positive integer k. A primitive Pythagorean triple is one in which a, b and c are pairwise coprime. A right triangle whose sides form a Pythagorean triple is called a Pythagorean triangle.
The name is derived from the Pythagorean theorem, stating that every right triangle has side lengths satisfying the formula a2 + b2 = c2; thus, Pythagorean triples describe the three integer side lengths of a right triangle. However, right triangles with non-integer sides do not form Pythagorean triples. For instance, the triangle with sides a = b = 1 and c = √2 is right, but (1, 1, √2) is not a Pythagorean triple because √2 is not an integer. Moreover, 1 and √2 do not have an integer common multiple because √2 is irrational. There are 16 primitive Pythagorean triples with c ≤ 100:
| ( 3 , 4 , 5 ) | ( 5, 12, 13) | ( 7, 24, 25) | ( 8, 15, 17) |
| ( 9, 40, 41) | (11, 60, 61) | (12, 35, 37) | (13, 84, 85) |
| (16, 63, 65) | (20, 21, 29) | (28, 45, 53) | (33, 56, 65) |
| (36, 77, 85) | (39, 80, 89) | (48, 55, 73) | (65, 72, 97) |
Each one of this low-c points forms one of the more easily-recognizable radiating lines in the scatter plot.

Contents |
Generating a triple

Euclid's formula[1] is a fundamental formula for generating Pythagorean triples given an arbitrary pair of positive integers m and n with m > n. The formula states that the integers
form a Pythagorean triple. The triple generated by Euclid's formula is primitive if and only if m and n are coprime and exactly one of them is even. If both n and m are odd, then a, b, and c will be even, and so the triple will not be primitive; however dividing  and
and  by 2 will yield a primitive triple if m and n are coprime and both odd.[2] Every primitive triple (possibly after exchanging a and b) arises from a unique pair of coprime numbers m, n, one of which is even. It follows that there are infinitely many primitive Pythagorean triples. This relationship of a and b to m and n from Euclid's formula is referenced throughout the rest of this article.
by 2 will yield a primitive triple if m and n are coprime and both odd.[2] Every primitive triple (possibly after exchanging a and b) arises from a unique pair of coprime numbers m, n, one of which is even. It follows that there are infinitely many primitive Pythagorean triples. This relationship of a and b to m and n from Euclid's formula is referenced throughout the rest of this article.
Despite generating all primitive triples, Euclid's formula does not produce all triples. This can be remedied by inserting an additional parameter k to the formula. The following will generate all Pythagorean triples (although not uniquely):
where m, n, and k are positive integers with m > n.
That these formulae generate Pythagorean triples can be verified by expanding a2 + b2 using elementary algebra and verifying that the result coincides with c2 (although this does not prove that it generates all such triples). Many other formulae for generating triples have been developed since the time of Euclid.
Elementary properties of primitive Pythagorean triples
The properties of a Primitive Pythagorean Triple (PPT) include:
- In a pythagorean triple,
- (c − a)(c − b)/2 is always a perfect square. This is particularly useful in checking if a given triple of numbers is a pythagorean triple, but it is only a necessary condition, not a sufficient one. The triple {6, 12, 18} passes (c − a)(c − b)/2 but is not a PPT. A simpler, more powerful test is, (by naming the even leg a): (c − a) and (c − b)/2 are both perfect squares. This is both necessary and sufficient for the triple to be a PT, but the PT may be derivative. If any two sides of a PT are relatively prime, it is a PPT.
- At most one of a, b, c is a square.
- There exist infinitely many primitive Pythagorean triples whose hypotenuses are squares of natural numbers.
- There exist infinitely many primitive Pythagorean triples in which one of the legs is the square of a natural number.
- The sum of the hypotenuse and the even leg of a primitive Pythagorean triple is the square of an odd number, and the arithmetic mean of the hypotenuse and the odd leg is a perfect square.
- The area (A = ab/2) is an even congruent number.
- Exactly one of a, b is odd; c is odd.
- Exactly one of a, b is divisible by 3.
- Exactly one of a, b is divisible by 4.
- Exactly one of a, b, c is divisible by 5.
- Exactly one of a, b, (a + b), (b − a) is divisible by 7.
- Exactly one of (a + c), (b + c), (c − a), (c − b) is divisible by 8.
- Exactly one of (a + c), (b + c), (c − a), (c − b) is divisible by 9.
- Exactly one of a, b, (2a + b), (2a − b), (2b + a), (2b − a) is divisible by 11.
- All prime factors of c are primes of the form 4n + 1.
- Every integer greater than 2 that is not congruent to 2 mod 4 is part of a primitive Pythagorean triple. In other words, integers greater than 2 which are not of the form 4n + 2.
- Every integer greater than 2 is part of a primitive or non-primitive Pythagorean triple, for example, the integers 6, 10, 14, and 18 are not part of primitive triples, but are part of the non-primitive triples 6, 8, 10; 14, 48, 50 and 18, 80, 82.
- There exist infinitely many Pythagorean triples in which the hypotenuse and the longer of the two legs differ by exactly one (such triples are necessarily primitive). Generalization: For every odd integer j, there exist infinitely many primitive Pythagorean triples in which the hypotenuse and the even leg differ by j 2.
- There exist infinitely many primitive Pythagorean triples in which the hypotenuse and the longer of the two legs differ by exactly two. Generalization: For every integer k > 0, there exist infinitely many primitive Pythagorean triples in which the hypotenuse and the odd leg differ by
 .
. - If j and k are odd positive integers, not necessarily unequal, there is exactly one primitive Pythagorean triple with
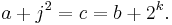
- The hypotenuse of every primitive Pythagorean triangle exceeds the even leg by the square of an odd integer j, and exceeds the odd leg by twice the square of an integer k > 0, from which it follows that:
- There are no primitive Pythagorean triples in which the hypotenuse and a leg differ by a prime number greater than 2.
- For each natural number n, there exist n Pythagorean triples with different hypotenuses and the same area.
- For each natural number n, there exist at least n different Pythagorean triples with the same leg a, where a is some natural number
- For each natural number n, there exist at least n different triangles with the same hypotenuse.
- In every Pythagorean triple, the radius of the incircle and the radii of the three excircles are natural numbers. (Actually the radius of the incircle can be shown to be
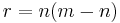 )
) - There are no Pythagorean triples in which the hypotenuse and one leg are the legs of another Pythagorean triple.[3]:p. 14
- The area of a Pythagorean triangle cannot be the square[3]:p. 17 or twice the square[3]:p. 21 of a natural number.
Some relationships

The radius, r, of the inscribed circle can be found by:
The unknown sides of a triple can be calculated directly from the radius of the incircle, r, and the value of a single known side, b.
- k = b − 2r
- a = 2r + (2 r2/k)
- c = a+ k = 2r + (2r2 /k) + k
The solution to the 'Incircle' problem shows that, for any circle whose radius is a whole number r, setting k = 1, we are guaranteed at least one right angled triangle containing this circle as its inscribed circle where the lengths of the sides of the triangle are a primitive Pythagorean triple:
- a=2r + 2r2
- b=2r + 1
- c=2r + 2r2 + 1
The perimeter P and area L of the right triangle corresponding to a primitive Pythagorean triple triangle are
- P = a + b + c = 2m(m + n)
- L = ab/2 = mn(m2 − n2)
Additional relationships:[4]
If two numbers of a triple are known, the third can be found using the Pythagorean theorem.
A special case: the Platonic sequence
The case n = 1 of the more general construction of Pythagorean triples has been known for a long time. Proclus, in his commentary to the 47th Proposition of the first book of Euclid's Elements, describes it as follows:
Certain methods for the discovery of triangles of this kind are handed down, one which they refer to Plato, and another to Pythagoras. (The latter) starts from odd numbers. For it makes the odd number the smaller of the sides about the right angle; then it takes the square of it, subtracts unity and makes half the difference the greater of the sides about the right angle; lastly it adds unity to this and so forms the remaining side, the hypotenuse.
...For the method of Plato argues from even numbers. It takes the given even number and makes it one of the sides about the right angle; then, bisecting this number and squaring the half, it adds unity to the square to form the hypotenuse, and subtracts unity from the square to form the other side about the right angle. ... Thus it has formed the same triangle that which was obtained by the other method.
In equation form, this becomes:
a is odd (Pythagoras, c. 540 BC):
a is even (Plato, c. 380 BC):
It can be shown that all Pythagorean triples are derivatives of the basic Platonic sequence (x,y,z) = p, (p2 - 1)/2 and (p2 + 1)/2 by allowing p to take non-integer rational values. If p is replaced with the rational fraction m/n in the sequence, the 'standard' triple generator 2mn, m2 - n2 and m2 + n2 results. It follows that every triple has a corresponding rational p value which can be used to generate a similar (i.e. equiangular) triangle with rational sides in the same proportion as the original. For example, the Platonic equivalent of (6, 8,10) is (3/2; 2, 5/2). The Platonic sequence itself can be derived by following the steps for 'splitting the square' described in Diophantus II.VIII.
Geometry of Euclid's formula
Euclid's formulae for a Pythagorean triple
can be understood in terms of the geometry of rational number points on the unit circle (Trautman 1998). To motivate this, consider a right triangle with legs a and b, and hypotenuse c, where a, b, and c are positive integers. By the Pythagorean theorem, a2 + b2 = c2 or, dividing both sides by c2,
Geometrically, the point in the Cartesian plane with coordinates
is on the unit circle x2 + y2 = 1. In this equation, the coordinates x and y are given by rational numbers. Conversely, any point on the unit circle whose coordinates x, y are rational numbers gives rise to a primitive Pythagorean triple. Indeed, write x and y as fractions in lowest terms:
where the greatest common divisor of a, b, and c is 1. Then, since x and y are on the unit circle,
as claimed.

There is therefore a correspondence between points on the unit circle with rational coordinates and primitive Pythagorean triples. At this point, Euclid's formulae can be derived either by methods of trigonometry or equivalently by using the stereographic projection.
For this, suppose that P′ is a point on the x-axis with rational coordinates P′(m/n,0). Then, it can be shown by basic algebra that the point P has coordinates
This establishes that each rational point of the x-axis goes over to a rational point of the unit circle. The converse, that every rational point of the unit circle comes from such a point of the x-axis, follows by applying the inverse stereographic projection. Suppose that P(x, y) is a point of the unit circle with x and y rational numbers. Then the point P′ obtained by stereographic projection onto the x-axis has coordinates
which is rational.
In terms of algebraic geometry, the algebraic variety of rational points on the unit circle is birational to the affine line over the rational numbers. The unit circle is thus called a rational curve, and it is this fact which enables an explicit parameterization of the (rational number) points on it by means of rational functions.
Spinors and the modular group
Pythagorean triples can likewise be encoded into a matrix of the form
A matrix of this form is symmetric. Furthermore, the determinant of X is
which is zero precisely when (a,b,c) is a Pythagorean triple. If X corresponds to a Pythagorean triple, then as a matrix it must have rank 1. Since X is symmetric, it follows from a result in linear algebra that there is a vector ξ = [m n]T such that the outer product
-
![X = 2\begin{bmatrix}m\\n\end{bmatrix}[m\ n] = 2\xi\xi^T\,](/2010-wikipedia_en_wp1-0.8_orig_2010-12/I/e28ce9aa533c131722e889c29ffe8cb6.png)
(
holds, where the T denotes the matrix transpose. The vector ξ is called a spinor (for the Lorentz group SO(1, 2)). In abstract terms, the Euclid formula means that each primitive Pythagorean triple can be written as the outer product with itself of a spinor with integer entries, as in (1).
The modular group Γ is the set of 2×2 matrices with integer entries
with determinant equal to one: αδ − βγ = 1. This set forms a group, since the inverse of a matrix in Γ is again in Γ, as is the product of two matrices in Γ. The modular group acts on the collection of all integer spinors. Furthermore, the group is transitive on the collection of integer spinors with relatively prime entries. For if [m n]T has relatively prime entries, then
where u and v are selected (by the Euclidean algorithm) so that mu + nv = 1.
By acting on the spinor ξ in (1), the action of Γ goes over to an action on Pythagorean triples, provided one allows for triples with possibly negative components. Thus if A is a matrix in Γ, then
-

(
gives rise to an action on the matrix X in (1). This does not give a well-defined action on primitive triples, since it may take a primitive triple to an imprimitive one. It is convenient at this point (per Trautman 1998) to call a triple (a,b,c) standard if c > 0 and either (a,b,c) are relatively prime or (a/2,b/2,c/2) are relatively prime with a/2 odd. If the spinor [m n]T has relatively prime entries, then the associated triple (a,b,c) determined by (1) is a standard triple. It follows that the action of the modular group is transitive on the set of standard triples.
Alternatively, restrict attention to those values of m and n for which m is odd and n is even. Let the subgroup Γ(2) of Γ be the kernel of the group homomorphism
where SL(2,Z2) is the special linear group over the finite field Z2 of integers modulo 2. Then Γ(2) is the group of unimodular transformations which preserve the parity of each entry. Thus if the first entry of ξ is odd and the second entry is even, then the same is true of Aξ for all A ∈ Γ(2). In fact, under the action (2), the group Γ(2) acts transitively on the collection of primitive Pythagorean triples (Alperin 2005).
The group Γ(2) is the free group whose generators are the matrices
Consequently, every primitive Pythagorean triple can be obtained in a unique way as a product of copies of the matrices U and L.
Parent/child relationships
By a result of Berggren (1934), all primitive Pythagorean triples can be generated from the (3, 4, 5) triangle by using the 3 linear transformations T1, T2, T3 below, where a, b, c are sides of a triple:
| new side a | new side b | new side c | |
| T1: | a − 2b + 2c | 2a − b + 2c | 2a − 2b + 3c |
| T2: | a + 2b + 2c | 2a + b + 2c | 2a + 2b + 3c |
| T3: | −a + 2b + 2c | −2a + b + 2c | −2a + 2b + 3c |
If one begins with 3, 4, 5 then all other primitive triples will eventually be produced. In other words, every primitive triple will be a “parent” to 3 additional primitive triples. Starting from the initial node with a = 3, b = 4, and c = 5, the next generation of triples is
| new side a | new side b | new side c |
| 3 − (2×4) + (2×5) = 5 | (2×3) − 4 + (2×5) = 12 | (2×3) − (2×4) + (3×5) = 13 |
| 3 + (2×4) + (2×5) = 21 | (2×3) + 4 + (2×5) = 20 | (2×3) + (2×4) + (3×5) = 29 |
| −3 + (2×4) + (2×5) = 15 | −(2×3) + 4 + (2×5) = 8 | −(2×3) + (2×4) + (3×5) = 17 |
The linear transformations T1, T2, and T3 have a geometric interpretation in the language of quadratic forms. They are closely related to (but are not equal to) reflections generating the orthogonal group of x2 + y2 − z2 over the integers.
For further discussion of parent-child relationships in triples, see: http://mathworld.wolfram.com/PythagoreanTriple.html and (Alperin 2005).
Relation to Gaussian integers
Alternatively, Euclid's formulae can be analyzed and proven using the Gaussian integers.[5] Gaussian integers are complex numbers of the form α = u + vi, where u and v are ordinary integers and i is the square root of negative one. The right-hand side of the Pythagorean theorem may be factored in Gaussian integers:
- c2 = a2 + b2 = (a + bi) (a − bi).
A primitive Pythagorean triple is one in which a and b are coprime, i.e., they share no prime factors in the integers. For such a triple, either a or b is even, and the other is odd; from this, it follows that c is also odd.
The two factors z := a + bi and z* := a − bi of a primitive Pythagorean triple each equal the square of a Gaussian integer. This can be proved using the property that every Gaussian integer can be factored uniquely into Gaussian primes up to units.[6] (This unique factorization follows from the fact that, roughly speaking, a version of the Euclidean algorithm can be defined on them.) The proof has three steps. First, if a and b share no prime factors in the integers, then they also share no prime factors in the Gaussian integers. (Assume a = gu and b = gv with Gaussian integers g, u and v and g not a unit. Then u and v lie on the same line through the origin. All Gaussian integers on such a line are integer multiples of some Gaussian integer h. But then the integer gh ≠ ±1 divides both a and b.) Second, it follows that z and z* likewise share no prime factors in the Gaussian integers. For if they did, then their common divisor δ would also divide z + z* = 2a and z - z* = 2ib. Since a and b are coprime, that implies that δ divides 2. From the formula c2 = zz*, that in turn would imply that c is even, contrary to the hypothesis of a primitive Pythagorean triple. Third, since c2 is a square, every Gaussian prime in its factorization is doubled, i.e., appears an even number of times. Since z and z* share no prime factors, this doubling is also true for them. Hence, z and z* are squares.
Thus, for example, the first factor can be written
- a + bi = (m + ni)2.
The real and imaginary parts of this equation give the two formulae
- a = m2 − n2,
- b = 2 mn.
For any primitive Pythagorean triple, there must be integers m and n such that these two equations are satisfied. Hence, every Pythagorean triple can be generated from some choice of these integers.
Distribution of triples

There are a number of results on the distribution of Pythagorean triples. In the scatter plot, a number of obvious patterns are already apparent. Whenever the legs (a,b) of a primitive triple appear in the plot, all integer multiples of (a,b) must also appear in the plot, and this property produces the appearance of lines radiating from the origin in the diagram.
Within the scatter, there are sets of parabolic patterns with a high density of points and all their foci at the origin, opening up in all four directions. Different parabolas intersect at the axes and appear to reflect off the axis with an incidence angle of 45 degrees, with a third parabola entering in a perpendicular fashion. Within this quadrant, each arc centered around the origin shows that section of the parabola that lies between its tip and its intersection with its semi-latus rectum.
These patterns can be explained as follows. If 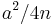 is an integer, then (a,
is an integer, then (a,  ,
,  ) is a Pythagorean triple. (In fact every Pythagorean triple (a, b, c) can be written in this way with integer n, possibly after exchanging a and b, since
) is a Pythagorean triple. (In fact every Pythagorean triple (a, b, c) can be written in this way with integer n, possibly after exchanging a and b, since 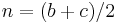 and a and b cannot both be odd.) The Pythagorean triples thus lie on curves given by
and a and b cannot both be odd.) The Pythagorean triples thus lie on curves given by  , that is, parabolas reflected at the a-axis, and the corresponding curves with a and b interchanged. If a is varied for a given n (i.e. on a given parabola), integer values of b occur relatively frequently if n is a square or a small multiple of a square. If several such values happen to lie close together, the corresponding parabolas approximately coincide, and the triples cluster in a narrow parabolic strip. For instance, 382 = 1444, 2 * 272 = 1458, 3 * 222 = 1452, 5 * 172 = 1445 and 10 * 122 = 1440; the corresponding parabolic strip around n ≈ 1450 is clearly visible in the scatter plot.
, that is, parabolas reflected at the a-axis, and the corresponding curves with a and b interchanged. If a is varied for a given n (i.e. on a given parabola), integer values of b occur relatively frequently if n is a square or a small multiple of a square. If several such values happen to lie close together, the corresponding parabolas approximately coincide, and the triples cluster in a narrow parabolic strip. For instance, 382 = 1444, 2 * 272 = 1458, 3 * 222 = 1452, 5 * 172 = 1445 and 10 * 122 = 1440; the corresponding parabolic strip around n ≈ 1450 is clearly visible in the scatter plot.
The angular properties described above follow immediately from the functional form of the parabolas. The parabolas are reflected at the a-axis at a = 2n, and the derivative of b with respect to a at this point is –1; hence the incidence angle is 45°. Since the clusters, like all triples, are repeated at integer multiples, the value 2n also corresponds to a cluster. The corresponding parabola intersects the b-axis at right angles at b = 2n, and hence it's reflection upon interchange of a and b intersects the a-axis at right angles at a = 2n, precisely where the parabola for n is reflected at the a-axis. (The same is of course true for a and b interchanged.)
Albert Fässler and others provide insights into the significance of these parabolas in the context of conformal mappings.[7][8]
Generalizations
There are several ways to generalize the concept of Pythagorean triples.
Pythagorean quadruple
A set of four positive integers a, b, c and d such that a2 + b2+ c2 = d2 is called a Pythagorean quadruple. The simplest example is (1, 2, 2, 3), since 12 + 22 + 22 = 32. The next simplest (primitive) example is (2, 3, 6, 7), since 22 + 32 + 62 = 72.
Quadruples are given by the formula
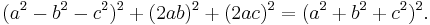
Pythagorean n-tuple
Using the simple algebraic identity,
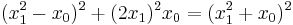
for arbitrary x0, x1, it is easy to prove that the square of the sum of n squares is itself the sum of n squares by letting x0 = x22+x32+...+xn2 and then distributing terms.[9] One can see how Pythagorean triples and quadruples are just the particular cases x0 = x22 and x0 = x22+x32, respectively, and so on for other n, with quintuples given by,
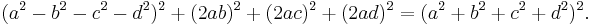
In addition, Pythagorean n-tuples with the first n–2 members being successive positive integers can be generated by[10]
where q=n–3 ≥ 0 and where
Fermat's Last Theorem
A generalization of the concept of Pythagorean triples is the search for triples of positive integers a, b, and c, such that an + bn = cn, for some n strictly greater than 2. Pierre de Fermat in 1637 claimed that no such triple exists, a claim that came to be known as Fermat's Last Theorem because it took longer than any other conjecture by Fermat to be proven or disproven. The first proof was given by Andrew Wiles in 1994.
n–1 or n nth powers summing to an nth power
Another generalization is searching for sets of n+1 positive integers for which the nth power of the last is the sum of the nth powers of the previous terms. The smallest sets for known values of n are:
- n=3: {3, 4, 5; 6}.
- n=4: {30, 120, 272, 315; 353}
- n=5: {19, 43, 46, 47, 67; 72}
- n=7: {127, 258, 266, 413, 430, 439, 525; 568}
- n=8: {90, 223, 478, 524, 748, 1088, 1190, 1324; 1409}
A slightly different generalization allows the sum of (k+1) nth powers to equal the sum of (n-k) nth powers. For example:
- (n=3): 13 + 123 = 93 + 103, made famous by Hardy's recollection of a conversation with Ramanujan about the number 1729 being the smallest number that can be expressed as a sum of two cubes in two distinct ways.
There can also exist n–1 positive integers whose nth powers sum to an nth power (though, by Fermat's last theorem, not for n=3); these are counterexamples to Euler's sum of powers conjecture. The smallest known counterexamples are
- n=4: (95800, 217519, 414560; 422481)
- n=5: (27, 84, 110, 133; 144)
Heronian triangle triples
A Heronian triangle is commonly defined as one with integer sides whose area is also an integer, and we shall consider Heronian triangles with distinct integer sides. The lengths of the sides of such a triangle form a Heronian triple (a, b, c) provided a < b < c. Clearly, any Pythagorean triple is a Heronian triple, since in a Pythagorean triple at least one of the legs a, b must be even, so that the area ab/2 is an integer. Not every Heronian triple is a Pythagorean triple, however, as the example (4, 13, 15) with area 24 shows.
If (a, b, c) is a Heronian triple, so is (ma, mb, mc) where m is any positive integer greater than one. The Heronian triple (a, b, c) is primitive provided a, b, c are pairwise relatively prime (as with a Pythagorean triple). Here are a few of the simplest primitive Heronian triples which are not Pythagorean triples:
- (4, 13, 15) with area 24
- (3, 25, 26) with area 36
- (7, 15, 20) with area 42
- (6, 25, 29) with area 60
- (11, 13, 20) with area 66
- (13, 14, 15) with area 84
- (13, 20, 21) with area 126
By Heron's formula, the extra condition for a triple of positive integers (a, b, c) with a < b < c to be Heronian is that
-
- (a2 + b2 + c2)2 - 2(a4 + b4 + c4)
or equivalently
-
- 2(a2b2 + a2c2 + b2c2) - (a4 + b4 + c4)
be a nonzero perfect square divisible by 16.
See also
- Heronian triangle
- Integer triangle
- Pythagorean prime
- Nonhypotenuse number
- Modular arithmetic
- Trigonometric identity
- Tangent half-angle formula
- Plimpton 322
- Diophantus II.VIII
- Hilbert's theorem 90
References
- ↑ "Euclid's Elements: Book X , Proposition XXIX". http://babbage.clarku.edu/~djoyce/java/elements/bookX/propX29.html.
- ↑ Mitchell, Douglas W., "An alternative characterisation of all primitive Pythagorean triples," Mathematical Gazette July 2001,.
- ↑ 3.0 3.1 3.2 Carmichael, R. D., 1914, "Diophantine analysis," in second half of R. D. Carmichael, The Theory of Numbers and Diophantine Analysis, Dover Publ., 1959.
- ↑ "Multiplying Pythagorean Triples". http://www.pythag.net/node9.html.
- ↑ *Stillwell J (2003), Elements of Number Theory, New York: Springer-Verlag, pp. 110–112, ISBN 0-387-95587-9 .
- ↑ Gauss CF (1832), "Theoria residuorum biquadraticorum", Comm. Soc. Reg. Sci. Gött. Rec. 4. See also Werke, 2:67–148.
- ↑ 1988 Preprint See Figure 2 on page 3., later published as Multiple Pythagorean number triples Albert Fässler, American Mathematical Monthly, v.98 n.6, pages 505-517, June/July 1991 ( 10.2307/2324870)
- ↑ Pythagorean triangles with legs less than n by Manuel Benito and Juan L. Varona, Journal of Computational and Applied Mathematics 143 (2002) 117–126
- ↑ "A Collection of Algebraic Identities: Sums of n Squares". http://sites.google.com/site/tpiezas/005b.
- ↑ Goehl, John F. Jr., "Reader reflections," Mathematics Teacher 98(9), May 2005, 580.
- Alperin, Roger C. (2005), "The modular tree of Pythagoras", American Mathematical Monthly (Mathematical Association of America) 112 (9): 807–816, doi:10.2307/30037602, MR2179860, http://www.math.sjsu.edu/~alperin/pt.pdf
- Berggren, B. (1934), "Pytagoreiska trianglar" (in Swedish), Tidskrift för elementär matematik, fysik och kemi 17: 129–139
- Barning, F.J.M. (1963), "Over pythagorese en bijna-pythagorese driehoeken en een generatieproces met behulp van unimodulaire matrices" (in Dutch), Math. Centrum Amsterdam Afd. Zuivere Wisk. ZW-011: 37, http://oai.cwi.nl/oai/asset/7151/7151A.pdf
- Eckert, Ernest (1992), "Primitive Pythagorean triples", The College Mathematics Journal (Mathematical Association of America) 23 (5): 413–417, doi:10.2307/2686417, http://www.jstor.org/stable/2686417
- Elkies, Noam, Pythagorean triples and Hilbert's theorem 90, http://www.math.harvard.edu/~elkies/Misc/hilbert.pdf.
- Heath, Thomas (1956), The Thirteen Books of Euclid's Elements Vol. 1 (Books I and II) (2nd ed.), Dover Publications, ISBN 0-486-60088-2.
- Martin, Artemas (1875), "Rational right angled triangles nearly isosceles", The Analyst (Annals of Mathematics) 3 (2): 47–50, doi:10.2307/2635906, http://www.jstor.org/pss/2635906
- McCullough, Darryl (2005), "Height and excess of Pythagorean triples", Mathematics Magazine 78 (1), http://www.math.ou.edu/~dmccullough/teaching/pythagoras2.pdf
- Romik, Dan (2004), The dynamics of Pythagorean triples, arXiv:math.DS/0406512
- Sierpinski, Wacław (2003), Pythagorean Triangles, Dover Publications, ISBN 0-486-43278-5.
- Teigen, M. G.; Hadwin, D. W. (1971), "On Generating Pythagorean Triples", The American Mathematical Monthly (Mathematical Association of America) 78 (4): 378–379, doi:10.2307/2316903, http://www.jstor.org/stable/2316903
- Trautman, Andrzej (1998), "Pythagorean spinors and Penrose twistors", in S.A. Hugget, L.J. Mason, K.P. Tod, S.T. Tsou, N.M.J. Woodhouse, Geometric universe, http://www.fuw.edu.pl/~amt/oxford.ps.
External links
- Weisstein, Eric W., "Pythagorean Triple" from MathWorld.
- Pythagorean Triples at cut-the-knot Interactive Applet showing unit circle relationships to Pythagorean Triples
- The Trinary Tree(s) underlying Primitive Pythagorean Triples at cut-the-knot
- Theoretical properties of the Pythagorean Triples and connections to geometry
- Clifford Algebras and Euclid's Parameterization of Pythagorean triples
- Pythagorean Triplets
- Discussion of Properties of Pythagorean triples, Interactive Calculators, Puzzles and Problems
- Generating Pythagorean Triples Using Arithmetic Progressions
- Parameterization of Pythagorean Triples by a single triple of polynomials
- Curious Consequences of a Miscopied Quadratic
- Solutions to Quadratic Compatible Pairs in relation to Pythagorean Triples
- The negative Pell equation and Pythagorean triples
- The Remarkable Incircle of a Triangle
- Interactive Calculator for Pythagorean Triples
- Price, H. Lee (2008), The Pythagorean Tree: A New Species, arXiv:0809.4324
- Pythagorean Triples and the Unit Circle, chap. 2-3, in "A Friendly Introduction to Number Theory" by Joseph H. Silverman, 3rd ed., 2006, Pearson Prentice Hall, Upper Saddle River, NJ, ISBN: 0-13-186137-9